3-46
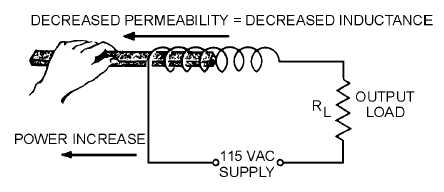
Earlier NEETS modules state that the inductance of a coil increases directly as the permeability of
the core material increases. If a coil is wound around an iron core, the permeability of the core is 5000.
Now, if the iron is pulled part way out of the coil of wire, the core is part iron and part air. The
permeability of the core decreases. As the permeability of the core decreases, the inductance of the coil
decreases. This increases the power delivered to the load (true power). This relationship is shown in
figure 3-30.
The system shown in figure 3-30 is not too practical. Even if a motor were used in place of the hand
that is shown, the resulting amplifier would be large, expensive, and not easily controlled. If the
permeability of a core could be changed by electrical means rather than mechanical, a more practical
system would result.
Figure 3-30.—Varying coil inductance with a movable coil.
High permeability depends on there being many molecular magnets (or electron spin directions) that
can be aligned to provide a path for magnetic lines of force. If almost all of these available paths are
already being used, the material is magnetized and there are no more paths for additional lines of force.
The "flux density" (number of lines of force passing through a given area) is as high as it can be. This
means that the permeability of the material has decreased. When this condition is reached, the core is said
to be SATURATED because it is saturated (filled) with all the magnetic lines of force it can pass. At this
point, the core has almost the same value of permeability as air (1) instead of the much higher value of
permeability (5000) that it had when it was unmagnetized.
Of course, the permeability does not suddenly change from 5000 to 1. The permeability changes as
the magnetizing force changes until saturation is reached. At saturation, permeability remains very low no
matter how much the magnetizing force increases. If you were to draw a graph of the flux density
compared to the magnetizing force, you would have something similar to the graph shown in figure 3-31.
Figure 3-31 also includes a curve representing the value of permeability as the magnetizing force
increases. Point "s" in figure 3-31 is the point of saturation. The flux density does not increase above
point "s," and the permeability is at a steady, low value.