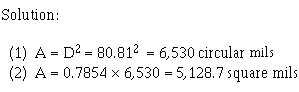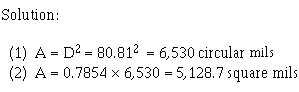1-3
square mils. The cross-sectional area of the wire has 625 circular mils but only 490.625 square mils.
Therefore, a circular mil represents a smaller unit of area than the square mil.
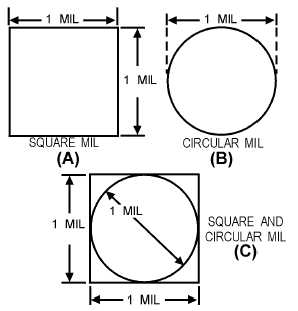
Figure 1-2.—A comparison of circular and square mils.
If a wire has a cross-sectional diameter of 1 mil, by definition, the circular mil area (CMA) is A =
D2, or A = 1
2, or A = 1 circular mil. To determine the square mil area of the same wire, apply the formula
A = pr2; therefore, A = 3.14 (.5)
2
(.5 representing half the diameter). When A = 3.14 .25, A = .7854
square mil. From this, it can be concluded that 1 circular mil is equal to. 7854 square mil. This becomes
important when square (view A of figure 1-2) and round (view B) conductors are compared as in view C
of figure 1-2.
When the square mil area is given, divide the area by 0.7854 to determine the circular mil area, or
CMA. When the CMA is given, multiply the area by 0.7854 to determine the square mil area. For
example,
Problem: A 12-gauge wire has a diameter of 80.81 mils. What is (1) its area in circular mils and (2)
its area in square mils?
Problem: A rectangular conductor is 1.5 inches wide and 0.25 inch thick. What is (1) its area in
square mils and (2) in circular mils? What size of round conductor is necessary to carry the same current
as the rectangular bar?