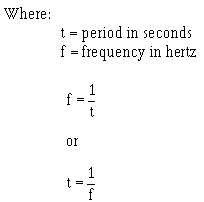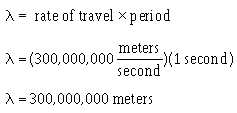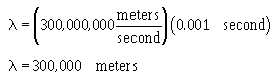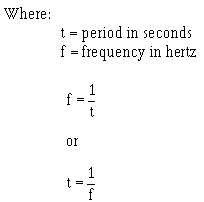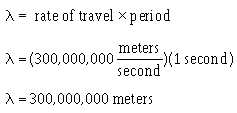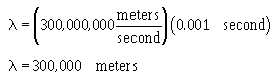1-8
WAVELENGTH
The WAVELENGTH of a sine wave is determined by its physical length. During the period a wave
is being generated, its leading edge is moving away from the source at 300,000,000 meters per second.
The physical length of the sine wave is determined by the amount of time it takes to complete one full
cycle. This wavelength is an important factor in determining the size of equipments used to generate and
transmit radio frequencies.
To help you understand the magnitude of the distance a wavefront (the initial part of a wave) travels
during 1 cycle, we will compute the wavelengths (l;) of several frequencies. Consider a vector that rotates
at 1 revolution per second. The resultant sine wave is transmitted into space by an antenna. As the vector
moves from its 0-degree starting position, the wavefront begins to travel away from the antenna. When
the vector reaches the 360-degree position, and the sine wave is completed, the sine wave is stretched out
over 300,000,000 meters. The reason the sine wave is stretched over such a great distance is that the
wavefront has been moving away from the antenna at 300,000,000 meters per second. This is shown in
the following example:
If a vector were rotating at 1,000 revolutions per second, its period would be 0.001 second. By
applying the formula for wavelength, you would find that the wavelength is 300,000 meters:
Since we normally know the frequency of a sine wave instead of its period, the wavelength is easier
to find using the frequency: