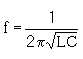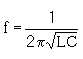1-6
X = XL
-
XC
Suppose an a.c. circuit contains an XL of 300 ohms and an XC of 250 ohms. The resultant reactance
is:
X = XL
-
XC = 300
-
250 = 50 ohms (inductive)
In some cases, the XC may be larger than the X
L. If XL = 1200 ohms and X
C
= 4000 ohms, the
difference is: X = XL
-
XC = 1200
-
4000 =
-
2800 ohms (capacitive). The total carries the sign (+ or
-
) of
the greater number (factor).
Q-1. What is the relationship between frequency and the values of (a) XL, (b) XC, and (c) R?
Q-2. In an a.c. circuit that contains both an inductor and a capacitor, what term is used for the
difference between the individual reactances?
RESONANCE
For every combination of L and C, there is only ONE frequency (in both series and parallel circuits)
that causes XL to exactly equal XC; this frequency is known as the RESONANT FREQUENCY. When
the resonant frequency is fed to a series or parallel circuit, X
L
becomes equal to XC, and the circuit is said
to be RESONANT to that frequency. The circuit is now called a RESONANT CIRCUIT; resonant
circuits are tuned circuits. The circuit condition wherein XL becomes equal to XC is known as
RESONANCE.
Each LCR circuit responds to resonant frequency differently than it does to any other frequency.
Because of this, an LCR circuit has the ability to separate frequencies. For example, suppose the TV or
radio station you want to see or hear is broadcasting at the resonant frequency. The LC "tuner" in your set
can divide the frequencies, picking out the resonant frequency and rejecting the other frequencies. Thus,
the tuner selects the station you want and rejects all other stations. If you decide to select another station,
you can change the frequency by tuning the resonant circuit to the desired frequency.
RESONANT FREQUENCY
As stated before, the frequency at which XL equals XC (in a given circuit) is known as the resonant
frequency of that circuit. Based on this, the following formula has been derived to find the exact resonant
frequency when the values of circuit components are known:
There are two important points to remember about this formula. First, the resonant frequency found
when using the formula will cause the reactances (XL and XC) of the L and C components to be equal.
Second, any change in the value of either L or C will cause a change in the resonant frequency.
An increase in the value of either L or C, or both L and C, will lower the resonant frequency of a
given circuit. A decrease in the value of L or C, or both L and C, will raise the resonant frequency of a
given circuit.