2-31
Figure 2-27C.—Overmodulation of a carrier. NONSINUSOIDAL MODULATING WAVE.
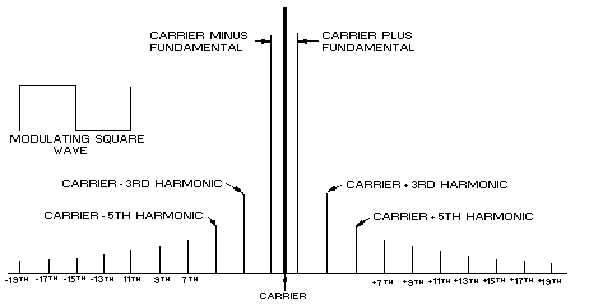
Observe the modulating square wave in figure 2-28. Remember that it contains an infinite number of
odd harmonics in addition to its fundamental frequency. Assume that a carrier has a frequency of 1
megahertz. The fundamental frequency of the modulating square wave is 1 kilohertz. When these signals
heterodyne, two new frequencies will be produced: a sum frequency of 1.001 megahertz and a difference
frequency of 0.999 megahertz. The fundamental frequency heterodynes with the carrier. This is also true
of all harmonics contained in the square wave. Side frequencies associated with those harmonics will be
produced as a result of this process. For example, the third harmonic of the square wave heterodynes with
the carrier and produces sideband frequencies at 1.003 and 0.997 megahertz. Another set will be produced
by the fifth, seventh, ninth, eleventh, thirteenth, fifteenth, seventeenth, and nineteenth harmonics of the
square wave, and so on to infinity.
Figure 2-28.—Spectrum distribution when modulating with a square wave.
Look at figure 2-28 and observe the relative amplitudes of the sidebands as they relate to the
amplitudes of the harmonics contained in the square wave. Note that the first set of sidebands is directly
related to the amplitude of the square wave. The second set of sidebands is related to the third harmonic
content of the square wave and is 1/3 the amplitude of the first set. The third set is related to the amplitude
of the first set of sidebands and is 115 the amplitude of the first set. This relationship will apply to each
additional set of sidebands.