2-3
In some cases, more than one variable is used in a single expression. For example, the expression
AB C D is spoken "A AND B AND NOT C AND D."
POSITIVE AND NEGATIVE LOGIC
To this point, we have been dealing with one type of LOGIC POLARITY, positive. Let’s further
define logic polarity and expand to cover in more detail the differences between positive and negative
logic.
Logic polarity is the type of voltage used to represent the logic 1 state of a statement. We have
determined that the two logic states can be represented by electrical signals. Any two distinct voltages
may be used. For instance, a positive voltage can represent the 1 state, and a negative voltage can
represent the 0 state. The opposite is also true.
Logic circuits are generally divided into two broad classes according to their polarity positive
logic and negative logic. The voltage levels used and a statement indicating the use of positive or negative
logic will usually be specified on logic diagrams supplied by manufacturers.
In practice, many variations of logic polarity are used; for example, from a high-positive to a low-
positive voltage, or from positive to ground; or from a high-negative to a low-negative voltage, or from
negative to ground. A brief discussion of the two general classes of logic polarity is presented in the
following paragraphs.
Positive Logic
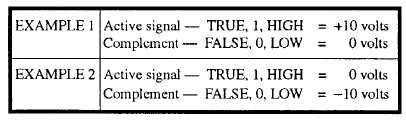
Positive logic is defined as follows: If the signal that activates the circuit (the 1 state) has a voltage
level that is more POSITIVE than the 0 state, then the logic polarity is considered to be POSITIVE. Table
2-2 shows the manner in which positive logic may be used.
Table 2-2. —Examples of Positive Logic
As you can see, in positive logic the 1 state is at a more positive voltage level than the 0 state.
Negative Logic
As you might suspect, negative logic is the opposite of positive logic and is defined as follows: If the
signal that activates the circuit (the 1 state) has a voltage level that is more NEGATIVE than the 0 state,
then the logic polarity is considered to be NEGATIVE. Table 2-3 shows the manner in which negative
logic may be used.